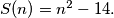Slični zadaci
For each positive integer  , let
, let  denote the number of ways of representing
denote the number of ways of representing  as a sum of powers of 2 with nonnegative integer exponents. Representations which differ only in the ordering of their summands are considered to be the same. For instance,
as a sum of powers of 2 with nonnegative integer exponents. Representations which differ only in the ordering of their summands are considered to be the same. For instance, 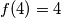 , because the number 4 can be represented in the following four ways: 4; 2+2; 2+1+1; 1+1+1+1.
, because the number 4 can be represented in the following four ways: 4; 2+2; 2+1+1; 1+1+1+1.
Prove that, for any integer we have
we have 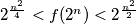 .
.
 , let
, let  denote the number of ways of representing
denote the number of ways of representing  as a sum of powers of 2 with nonnegative integer exponents. Representations which differ only in the ordering of their summands are considered to be the same. For instance,
as a sum of powers of 2 with nonnegative integer exponents. Representations which differ only in the ordering of their summands are considered to be the same. For instance, 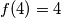 , because the number 4 can be represented in the following four ways: 4; 2+2; 2+1+1; 1+1+1+1.
, because the number 4 can be represented in the following four ways: 4; 2+2; 2+1+1; 1+1+1+1. Prove that, for any integer
 we have
we have 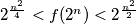 .
.  Školjka
Školjka  be the length of the longest of these segments. We define a diameter of the set to be any connecting segment of length
be the length of the longest of these segments. We define a diameter of the set to be any connecting segment of length  be an integer. Prove that if
be an integer. Prove that if 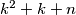 is prime for all integers
is prime for all integers  such that
such that 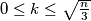 , then
, then 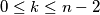 .(IMO Problem 6)
.(IMO Problem 6)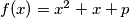 ,
,  Prove that if the numbers
Prove that if the numbers 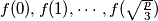 are primes, then all the numbers
are primes, then all the numbers 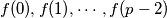 are primes.
are primes. and
and  be two positive integers such that
be two positive integers such that 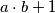 divides
divides 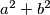 . Show that
. Show that 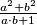 is a perfect square.
is a perfect square. 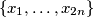 of the set
of the set 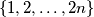 where
where  if
if 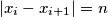 for at least one
for at least one  in
in 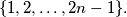 Show that, for each
Show that, for each  on the real line
on the real line 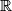 superinvariant if for any stretching
superinvariant if for any stretching  of the set by the transformation taking
of the set by the transformation taking  to
to 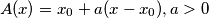 there exists a translation
there exists a translation 
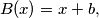 such that the images of
such that the images of  agree; i.e., for any
agree; i.e., for any 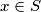 there is a
there is a 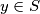 such that
such that  and for any
and for any 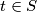 there is a
there is a 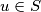 such that
such that 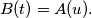 Determine all superinvariant sets.
Determine all superinvariant sets. 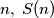 is defined to be the greatest integer such that, for every positive integer
is defined to be the greatest integer such that, for every positive integer 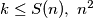 can be written as the sum of
can be written as the sum of 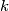 positive squares.
positive squares. 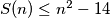 for each
for each 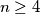 .
. 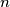 such that
such that  .
.