Find all functions
defined for all
that satisfy the condition
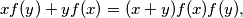
for all
and
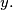
Prove that exactly two of them are continuous.
%V0
$(BUL 2)$ Find all functions $f$ defined for all $x$ that satisfy the condition $xf(y) + yf(x) = (x + y)f(x)f(y),$ for all $x$ and $y.$ Prove that exactly two of them are continuous.
Školjka