Slični zadaci
We consider a prism which has the upper and inferior basis the pentagons:  and
and 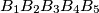 . Each of the sides of the two pentagons and the segments
. Each of the sides of the two pentagons and the segments 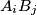 with
with 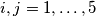 is colored in red or blue. In every triangle which has all sides colored there exists one red side and one blue side. Prove that all the 10 sides of the two basis are colored in the same color.
is colored in red or blue. In every triangle which has all sides colored there exists one red side and one blue side. Prove that all the 10 sides of the two basis are colored in the same color.
 and
and 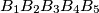 . Each of the sides of the two pentagons and the segments
. Each of the sides of the two pentagons and the segments 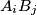 with
with 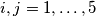 is colored in red or blue. In every triangle which has all sides colored there exists one red side and one blue side. Prove that all the 10 sides of the two basis are colored in the same color.
is colored in red or blue. In every triangle which has all sides colored there exists one red side and one blue side. Prove that all the 10 sides of the two basis are colored in the same color.  Školjka
Školjka  in the plane of the triangle
in the plane of the triangle 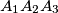 . Define
. Define 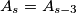 for all
for all 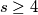 . Construct a set of points
. Construct a set of points 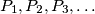 such that
such that 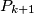 is the image of
is the image of  under a rotation center
under a rotation center 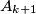 through an angle
through an angle 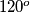 clockwise for
clockwise for 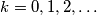 . Prove that if
. Prove that if 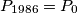 , then the triangle
, then the triangle  is
is 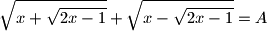 given
given  ;
; 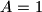 ;
;  ,
,  ,
,  ,
,  be the sides of a triangle, and
be the sides of a triangle, and  its area. Prove:
its area. Prove: 
 be the lengths of the sides of a triangle, and
be the lengths of the sides of a triangle, and 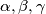 respectively, the angles opposite these sides. Prove that if
respectively, the angles opposite these sides. Prove that if 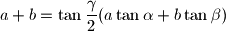 the triangle is isosceles.
the triangle is isosceles.  let
let  be the altitude drawn to the hypotenuse and let the straight line joining the incentres of the triangles
be the altitude drawn to the hypotenuse and let the straight line joining the incentres of the triangles 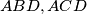 intersect the sides
intersect the sides 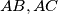 at the points
at the points 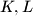 respectively. If
respectively. If  and
and 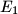 dnote the areas of triangles
dnote the areas of triangles 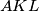 respectively, show that
respectively, show that 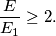
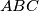 be a triangle and
be a triangle and 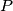 an interior point of
an interior point of  is less than or equal to
is less than or equal to 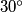 .
.