Slični zadaci
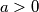 and
and  ,
,  are integers such that
are integers such that  –
– 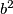 is a square-free positive integer P. For example P could be 3*5, but not 3^2*5. Let
is a square-free positive integer P. For example P could be 3*5, but not 3^2*5. Let  be the number of pairs of integers
be the number of pairs of integers 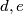 such that
such that  . Show that
. Show that is finite and that
is finite and that 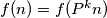 for every positive integer
for every positive integer  .
. Original Statement:
Let
 be given integers
be given integers 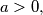
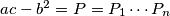 where
where 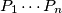 are (distinct) prime numbers. Let
are (distinct) prime numbers. Let 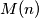 denote the number of pairs of integers
denote the number of pairs of integers 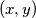 for which
for which 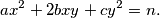 Prove that
Prove that 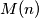 is finite and
is finite and 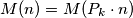 for every integer
for every integer 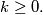 Note that the "
Note that the " " in
" in 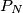 and the "
and the " " in
" in 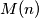 do not have to be the same.
do not have to be the same.  Školjka
Školjka 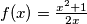 for
for 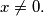 Define
Define 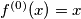 and
and 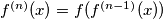 for all positive integers
for all positive integers 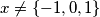
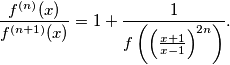
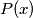 be a non-constant polynomial with integer coefficients. Prove that there is no function
be a non-constant polynomial with integer coefficients. Prove that there is no function  from the set of integers into the set of integers such that the number of integers
from the set of integers into the set of integers such that the number of integers  with
with 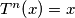 is equal to
is equal to 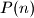 for every
for every 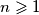 , where
, where  denotes the
denotes the  let
let 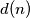 denote the number of (positive) divisors of
denote the number of (positive) divisors of 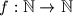 with the following properties:
with the following properties:  for all
for all  .
. 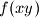 divides
divides 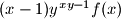 for all
for all 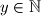 .
. 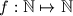 such that for every
such that for every 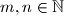 and every prime
and every prime  the number
the number 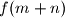 is divisible by
is divisible by  if and only if
if and only if 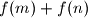 is divisible by
is divisible by 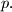
 be a non-empty set of positive integers. Suppose that there are positive integers
be a non-empty set of positive integers. Suppose that there are positive integers 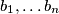 and
and 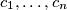 such that
such that  the set
the set  is a subset of
is a subset of 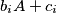 and
and 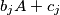 are disjoint whenever
are disjoint whenever 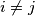

![a=\root3\of n\kern1.5pt,\qquad b={1\over a-[a]}\kern1pt,\qquad c={1\over b-[b]}\kern1.5pt,](/media/m/8/2/d/82d50c7f89d4ecc16f6afad9981c6d07.png)
![[x]](/media/m/6/a/4/6a47dfb91475b9d5490dbb3a666604a3.png) denotes the integer part of
denotes the integer part of 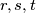 , not all zero, such that
, not all zero, such that 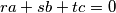 .
.