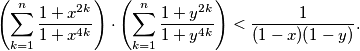Slični zadaci
A game is played by  girls (
girls ( ), everybody having a ball. Each of the
), everybody having a ball. Each of the 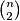 pairs of players, is an arbitrary order, exchange the balls they have at the moment. The game is called nice nice if at the end nobody has her own ball and it is called tiresome if at the end everybody has her initial ball. Determine the values of
pairs of players, is an arbitrary order, exchange the balls they have at the moment. The game is called nice nice if at the end nobody has her own ball and it is called tiresome if at the end everybody has her initial ball. Determine the values of  for which there exists a nice game and those for which there exists a tiresome game.
for which there exists a nice game and those for which there exists a tiresome game.
 girls (
girls ( ), everybody having a ball. Each of the
), everybody having a ball. Each of the 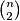 pairs of players, is an arbitrary order, exchange the balls they have at the moment. The game is called nice nice if at the end nobody has her own ball and it is called tiresome if at the end everybody has her initial ball. Determine the values of
pairs of players, is an arbitrary order, exchange the balls they have at the moment. The game is called nice nice if at the end nobody has her own ball and it is called tiresome if at the end everybody has her initial ball. Determine the values of  for which there exists a nice game and those for which there exists a tiresome game.
for which there exists a nice game and those for which there exists a tiresome game. For  and
and 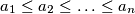 given real numbers we have the following instructions:
given real numbers we have the following instructions:
- place out the numbers in some order in a ring;
- delete one of the numbers from the ring;
- if just two numbers are remaining in the ring: let be the sum of these two numbers. Otherwise, if there are more the two numbers in the ring, replace
be the sum of these two numbers. Otherwise, if there are more the two numbers in the ring, replace
Afterwards start again with the step (2). Show that the largest sum which can result in this way is given by the formula
which can result in this way is given by the formula
![S_{max}= \sum^n_{k=2} \begin{pmatrix} n -2 \\ [\frac{k}{2}] - 1\end{pmatrix}a_{k}.](/media/m/a/d/4/ad4e65bc42b08da06d18502a103d6a04.png)
 and
and 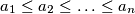 given real numbers we have the following instructions:
given real numbers we have the following instructions: - place out the numbers in some order in a ring;
- delete one of the numbers from the ring;
- if just two numbers are remaining in the ring: let
 be the sum of these two numbers. Otherwise, if there are more the two numbers in the ring, replace
be the sum of these two numbers. Otherwise, if there are more the two numbers in the ring, replace Afterwards start again with the step (2). Show that the largest sum
 which can result in this way is given by the formula
which can result in this way is given by the formula ![S_{max}= \sum^n_{k=2} \begin{pmatrix} n -2 \\ [\frac{k}{2}] - 1\end{pmatrix}a_{k}.](/media/m/a/d/4/ad4e65bc42b08da06d18502a103d6a04.png)
 Školjka
Školjka  be the real polynomial function,
be the real polynomial function,  Prove that if
Prove that if 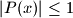 for all
for all  such that
such that 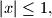 then
then 
 be non-negative reals, not all zero. Show that that
be non-negative reals, not all zero. Show that that 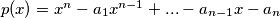 has preceisely 1 positive real root
has preceisely 1 positive real root  .
.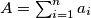 and
and 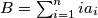 . Show that
. Show that 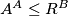 .
.  and
and  be positive real numbers such that
be positive real numbers such that  . Prove that
. Prove that 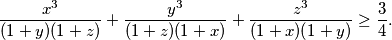
 are three positive real numbers such that
are three positive real numbers such that 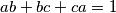 , prove that
, prove that ![\sqrt[3]{ \frac{1}{a} + 6b} + \sqrt[3]{\frac{1}{b} + 6c} + \sqrt[3]{\frac{1}{c} + 6a } \leq \frac{1}{abc}.](/media/m/b/8/1/b8152e5174c814f5f157b14f7eb88b60.png)
 be a positive real number such that
be a positive real number such that 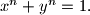 Prove that
Prove that