The lengths of the sides of a convex hexagon
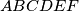
satisfy
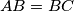
,
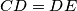
,
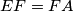
. Prove that:
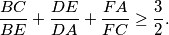
%V0
The lengths of the sides of a convex hexagon $ABCDEF$ satisfy $AB = BC$, $CD = DE$, $EF = FA$. Prove that:
$$\frac {BC}{BE} + \frac {DE}{DA} + \frac {FA}{FC} \geq \frac {3}{2}.$$