Given a triangle

. The points

,

,

divide the circumcircle

of the triangle

into three arcs

,

,

. Let

be a variable point on the arc

, and let
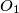
and

be the incenters of the triangles
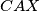
and
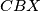
. Prove that the circumcircle of the triangle
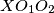
intersects the circle

in a fixed point.
%V0
Given a triangle $ABC$. The points $A$, $B$, $C$ divide the circumcircle $\Omega$ of the triangle $ABC$ into three arcs $BC$, $CA$, $AB$. Let $X$ be a variable point on the arc $AB$, and let $O_{1}$ and $O_{2}$ be the incenters of the triangles $CAX$ and $CBX$. Prove that the circumcircle of the triangle $XO_{1}O_{2}$ intersects the circle $\Omega$ in a fixed point.