Let
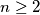
be a positive integer, with divisors
. Prove that
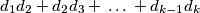
is always less than
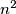
, and determine when it is a divisor of
.
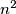
%V0
Let $n\geq2$ be a positive integer, with divisors $1=d_1<d_2<\,\ldots<d_k=n$. Prove that $d_1d_2+d_2d_3+\,\ldots\,+d_{k-1}d_k$ is always less than $n^2$, and determine when it is a divisor of $n^2$.
Školjka 
of integers such that 
such that p is a prime, 
and 
is a divisor of 
. 
of positive integers such that 
is divisible by 
. 
and 
are such that the numbers 
and 
are both squares of positive integers. What is the least possible value that can be taken on by the smaller of these two squares? 
where 
and 
are positive integers such that 
is an integer.