Given a triangle

satisfying
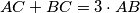
. The incircle of triangle

has center

and touches the sides

and

at the points

and

, respectively. Let

and

be the reflections of the points

and

with respect to

. Prove that the points

,

,

,

lie on one circle.
%V0
Given a triangle $ABC$ satisfying $AC+BC=3\cdot AB$. The incircle of triangle $ABC$ has center $I$ and touches the sides $BC$ and $CA$ at the points $D$ and $E$, respectively. Let $K$ and $L$ be the reflections of the points $D$ and $E$ with respect to $I$. Prove that the points $A$, $B$, $K$, $L$ lie on one circle.