Let  be a fixed convex quadrilateral with
be a fixed convex quadrilateral with 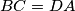 and
and  not parallel with
not parallel with  . Let two variable points
. Let two variable points  and
and  lie of the sides
lie of the sides  and
and  , respectively and satisfy
, respectively and satisfy 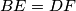 . The lines
. The lines  and
and  meet at
meet at  , the lines
, the lines  and
and  meet at
meet at  , the lines
, the lines  and
and  meet at
meet at  .
.
Prove that the circumcircles of the triangles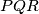 , as
, as  and
and  vary, have a common point other than
vary, have a common point other than  .
.
 be a fixed convex quadrilateral with
be a fixed convex quadrilateral with 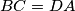 and
and  not parallel with
not parallel with  . Let two variable points
. Let two variable points  and
and  lie of the sides
lie of the sides  and
and  , respectively and satisfy
, respectively and satisfy 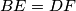 . The lines
. The lines  and
and  meet at
meet at  , the lines
, the lines  and
and  meet at
meet at  , the lines
, the lines  and
and  meet at
meet at  .
. Prove that the circumcircles of the triangles
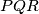 , as
, as  and
and  vary, have a common point other than
vary, have a common point other than  .
.  Školjka
Školjka