Let
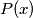
be a non-constant polynomial with integer coefficients. Prove that there is no function

from the set of integers into the set of integers such that the number of integers

with
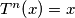
is equal to
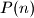
for every
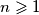
, where

denotes the

-fold application of

.
Proposed by Jozsef Pelikan, Hungary
%V0
Let $P\!\left(x\right)$ be a non-constant polynomial with integer coefficients. Prove that there is no function $T$ from the set of integers into the set of integers such that the number of integers $x$ with $T^n\!\left(x\right) = x$ is equal to $P\!\left(n\right)$ for every $n \geqslant 1$, where $T^n$ denotes the $n$-fold application of $T$.
Proposed by Jozsef Pelikan, Hungary