Slični zadaci
Let 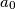 ,
,  ,
, 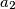 , ... be an infinite sequence of real numbers satisfying the equation
, ... be an infinite sequence of real numbers satisfying the equation 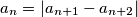 for all
for all 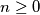 , where
, where 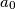 and
and  are two different positive reals.
are two different positive reals.
Can this sequence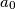 ,
,  ,
, 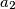 , ... be bounded?
, ... be bounded?
Remark This one is from the IMO Shortlist 2004, but it's already published on the official BWM website und thus I take the freedom to post it here:
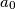 ,
,  ,
, 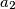 , ... be an infinite sequence of real numbers satisfying the equation
, ... be an infinite sequence of real numbers satisfying the equation 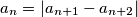 for all
for all 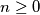 , where
, where 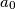 and
and  are two different positive reals.
are two different positive reals. Can this sequence
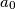 ,
,  ,
, 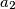 , ... be bounded?
, ... be bounded? Remark This one is from the IMO Shortlist 2004, but it's already published on the official BWM website und thus I take the freedom to post it here:
 Školjka
Školjka 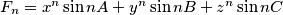 , gdje su
, gdje su 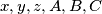 realni brojevi takvi da je
realni brojevi takvi da je 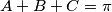 . Ako je
. Ako je 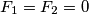 , dokažite da je
, dokažite da je  za svaki prirodni broj
za svaki prirodni broj  .
. 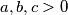 such that
such that 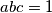 . Prove that
. Prove that 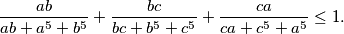
 be non-negative reals, not all zero. Show that that
be non-negative reals, not all zero. Show that that 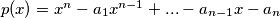 has preceisely 1 positive real root
has preceisely 1 positive real root  .
.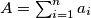 and
and 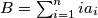 . Show that
. Show that 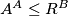 .
. 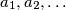 be an infinite sequence of real numbers, for which there exists a real number
be an infinite sequence of real numbers, for which there exists a real number  with
with 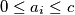 for all
for all  , such that
, such that 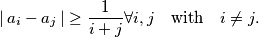
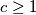 .
.  is a sequence of real numbers such that
is a sequence of real numbers such that ![a_{n + 1} = \left[a_{n}\right]\cdot \left\{a_{n}\right\}](/media/m/8/4/1/841a9a2ad06c20187088b2f582b7c6d1.png)
 such that for every
such that for every  we have
we have 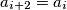 .
. 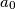 ,
, 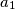 ,
, 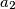 ,
,  be a sequence of reals such that
be a sequence of reals such that 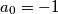 and
and 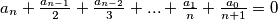 for all
for all 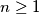 .
. 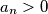 for all
for all