Let
and
be two positive integers. Let
,
,
,
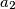
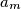
be
different numbers from the set
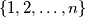
such that for any two indices
and
with
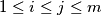
and
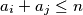
, there exists an index
such that
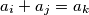
. Show that
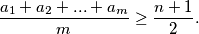
%V0
Let $m$ and $n$ be two positive integers. Let $a_1$, $a_2$, $\ldots$, $a_m$ be $m$ different numbers from the set $\{1, 2,\ldots, n\}$ such that for any two indices $i$ and $j$ with $1\leq i \leq j \leq m$ and $a_i + a_j \leq n$, there exists an index $k$ such that $a_i + a_j = a_k$. Show that
$$\frac {a_1 + a_2 + ... + a_m}{m} \geq \frac {n + 1}{2}.$$
Školjka