Slični zadaci
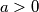 and
and  ,
,  are integers such that
are integers such that  –
– 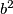 is a square-free positive integer P. For example P could be 3*5, but not 3^2*5. Let
is a square-free positive integer P. For example P could be 3*5, but not 3^2*5. Let  be the number of pairs of integers
be the number of pairs of integers 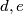 such that
such that  . Show that
. Show that is finite and that
is finite and that 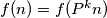 for every positive integer
for every positive integer  .
. Original Statement:
Let
 be given integers
be given integers 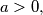
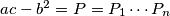 where
where 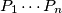 are (distinct) prime numbers. Let
are (distinct) prime numbers. Let 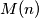 denote the number of pairs of integers
denote the number of pairs of integers 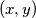 for which
for which 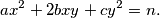 Prove that
Prove that 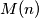 is finite and
is finite and 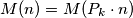 for every integer
for every integer 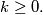 Note that the "
Note that the " " in
" in 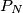 and the "
and the " " in
" in 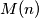 do not have to be the same.
do not have to be the same. Let  be the set of all pairs
be the set of all pairs 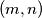 of relatively prime positive integers
of relatively prime positive integers  with
with  even and
even and  For
For 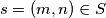 write
write  where
where 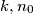 are positive integers with
are positive integers with  odd and define
odd and define  Prove that
Prove that  is a function from
is a function from  to
to  and that for each
and that for each 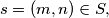 there exists a positive integer
there exists a positive integer 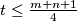 such that
such that 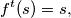 where
where 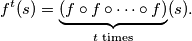
If is a prime number which does not divide
is a prime number which does not divide 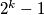 for
for 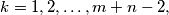 prove that the smallest value
prove that the smallest value  which satisfies the above conditions is
which satisfies the above conditions is ![\left [\frac{m+n+1}{4} \right ]](/media/m/8/7/8/878d7261cbd40fe8c838d36d2b94fea7.png) where
where ![\left[ x \right]](/media/m/8/4/7/847a3b7449538c2b99179a2953e7f9e0.png) denotes the greatest integer
denotes the greatest integer 
 be the set of all pairs
be the set of all pairs 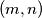 of relatively prime positive integers
of relatively prime positive integers  with
with  even and
even and  For
For 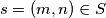 write
write  where
where 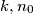 are positive integers with
are positive integers with  odd and define
odd and define  Prove that
Prove that  is a function from
is a function from  to
to  and that for each
and that for each 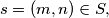 there exists a positive integer
there exists a positive integer 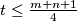 such that
such that 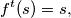 where
where 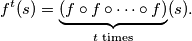
If
 is a prime number which does not divide
is a prime number which does not divide 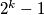 for
for 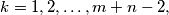 prove that the smallest value
prove that the smallest value  which satisfies the above conditions is
which satisfies the above conditions is ![\left [\frac{m+n+1}{4} \right ]](/media/m/8/7/8/878d7261cbd40fe8c838d36d2b94fea7.png) where
where ![\left[ x \right]](/media/m/8/4/7/847a3b7449538c2b99179a2953e7f9e0.png) denotes the greatest integer
denotes the greatest integer 
 Školjka
Školjka  are three positive real numbers such that
are three positive real numbers such that 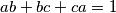 , prove that
, prove that ![\sqrt[3]{ \frac{1}{a} + 6b} + \sqrt[3]{\frac{1}{b} + 6c} + \sqrt[3]{\frac{1}{c} + 6a } \leq \frac{1}{abc}.](/media/m/b/8/1/b8152e5174c814f5f157b14f7eb88b60.png)
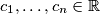 with
with  such that
such that 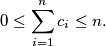 Show that we can find integers
Show that we can find integers 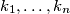 such that
such that 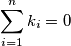 and
and 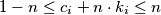 for every
for every 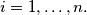
 with
with  Show that there exist integers
Show that there exist integers  and
and 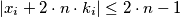 for every
for every 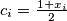 for
for 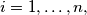 etc.
etc. 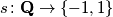 such that if
such that if  and
and  are distinct rational numbers satisfying
are distinct rational numbers satisfying 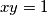 or
or  , then
, then 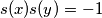 ? Justify your answer.
? Justify your answer. 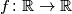 satisfying the equation
satisfying the equation 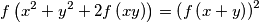 for all
for all 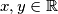 .
. 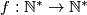 satisfying
satisfying 
 and
and 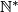 stands for the set of all positive integers:
stands for the set of all positive integers: 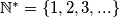 .
. 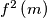 , we mean
, we mean 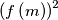 (and not
(and not  ).
).