Let  be a positive integer. Prove that the number
be a positive integer. Prove that the number 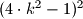 has a positive divisor of the form
has a positive divisor of the form  if and only if
if and only if  is even.
is even.
Actual IMO 2007 Problem, posed as question 5 in the contest, which was used as a lemma in the official solutions for problem N6 as shown above.
Author: Kevin Buzzard and Edward Crane, United Kingdom
 be a positive integer. Prove that the number
be a positive integer. Prove that the number 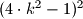 has a positive divisor of the form
has a positive divisor of the form  if and only if
if and only if  is even.
is even. Actual IMO 2007 Problem, posed as question 5 in the contest, which was used as a lemma in the official solutions for problem N6 as shown above.
Author: Kevin Buzzard and Edward Crane, United Kingdom
 Školjka
Školjka  such that
such that 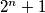 ?
? 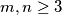 for which there exist infinitely many positive integers
for which there exist infinitely many positive integers  such that
such that  is itself an integer.
is itself an integer.  be a prime number. Prove that there exists a prime number
be a prime number. Prove that there exists a prime number  such that for every integer
such that for every integer  is not divisible by
is not divisible by  be a polynomial of degree
be a polynomial of degree 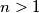 with integer coefficients and let
with integer coefficients and let 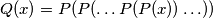 , where
, where  occurs
occurs  such that
such that 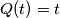 .
.  has a prime divisor greater than
has a prime divisor greater than 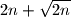 .
.