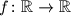
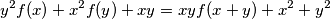
Find all functions such that the equality holds for all , where is the set of real numbers.
%V0
Find all functions $f \colon \mathbb R \to \mathbb R$ such that the equality $$y^2f(x) + x^2f(y) + xy = xyf(x + y) + x^2 + y^2$$ holds for all $x, y \in \Bbb R$, where $\Bbb R$ is the set of real numbers.
Izvor: Srednjoeuropska matematička olimpijada 2011, ekipno natjecanje, problem 1
Školjka 
such that the equality 
holds for all 
, where 
is the set of real numbers.