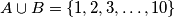
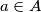
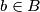
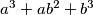
Let and be disjoint nonempty sets with . Show that there exist elements and such that the number is divisible by .
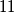
%V0
Let $A$ and $B$ be disjoint nonempty sets with $A \cup B = \{1, 2,3, \ldots, 10\}$. Show that there exist elements $a \in A$ and $b \in B$ such that the number $a^3 + ab^2 + b^3$ is divisible by $11$.
Izvor: Srednjoeuropska matematička olimpijada 2011, ekipno natjecanje, problem 7
Školjka 
and 
be disjoint nonempty sets with 
. Show that there exist elements 
and 
such that the number 
is divisible by 
.