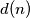
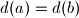
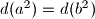
For any positive integer let denote the number of positive divisors of . Do there exist positive integers and , such that and , but ?
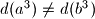
%V0
For any positive integer $n$ let $d(n)$ denote the number of positive divisors of $n$. Do there exist positive integers $a$ and $b$, such that $d(a)=d(b)$ and $d(a^2 ) = d(b^2 )$, but $d(a^3 ) \ne d(b^3 )$ ?
Izvor: Srednjoeuropska matematička olimpijada 2012, ekipno natjecanje, problem 8
Školjka 
let 
denote the number of positive divisors of 
and 
, such that 
and 
, but 
?