Let

denote the set of all ordered triples
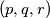
of nonnegative integers. Find all functions
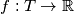
satisfying
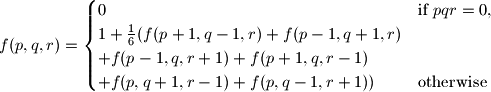
for all nonnegative integers

,

,

.
%V0
Let $T$ denote the set of all ordered triples $\left(p,q,r\right)$ of nonnegative integers. Find all functions $f: T \rightarrow \mathbb{R}$ satisfying
$$f(p,q,r) =
\begin{cases}
0 &\text{if}\; pqr = 0,\\
1+\frac{1}{6}(f(p+1,q-1,r)+f(p-1,q+1,r) &\\
+f(p-1,q,r+1)+f(p+1,q,r-1) &\\
+f(p,q+1,r-1)+f(p,q-1,r+1)) &\text{otherwise}\end{cases}$$
for all nonnegative integers $p$, $q$, $r$.