Let  be an integer. Consider an
be an integer. Consider an  chessboard consisting of
chessboard consisting of 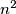 unit squares. A configuration of
unit squares. A configuration of  rooks on this board is peaceful if every row and every column contains exactly one rook. Find the greatest positive integer
rooks on this board is peaceful if every row and every column contains exactly one rook. Find the greatest positive integer  such that, for each peaceful configuration of
such that, for each peaceful configuration of  rooks, there is a
rooks, there is a 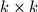 square which does not contain a rook on any of its
square which does not contain a rook on any of its 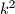 unit squares.
unit squares.
 be an integer. Consider an
be an integer. Consider an  chessboard consisting of
chessboard consisting of 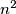 unit squares. A configuration of
unit squares. A configuration of  rooks on this board is peaceful if every row and every column contains exactly one rook. Find the greatest positive integer
rooks on this board is peaceful if every row and every column contains exactly one rook. Find the greatest positive integer  such that, for each peaceful configuration of
such that, for each peaceful configuration of  rooks, there is a
rooks, there is a 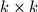 square which does not contain a rook on any of its
square which does not contain a rook on any of its 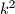 unit squares.
unit squares. For each positive integer  , the Bank of Cape Town issues coins of denomination
, the Bank of Cape Town issues coins of denomination 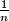 . Given a finite collection of such coins (of not necessarily different denominations) with total value at most
. Given a finite collection of such coins (of not necessarily different denominations) with total value at most 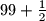 , prove that it is possible to split this collection into
, prove that it is possible to split this collection into 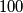 or fewer groups, such that each group has total value at most
or fewer groups, such that each group has total value at most  .
.
 , the Bank of Cape Town issues coins of denomination
, the Bank of Cape Town issues coins of denomination 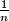 . Given a finite collection of such coins (of not necessarily different denominations) with total value at most
. Given a finite collection of such coins (of not necessarily different denominations) with total value at most 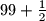 , prove that it is possible to split this collection into
, prove that it is possible to split this collection into 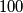 or fewer groups, such that each group has total value at most
or fewer groups, such that each group has total value at most  .
. A set of line sin the plane is in general position if no two are parallel and no three pass through the same point. A set of lines in general position cuts the plane into regions, some of which have finite area; we call these its finite regions. Prove that for all sufficiently large  , in any set of
, in any set of  lines in general position it is possible to colour at least
lines in general position it is possible to colour at least 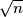 of the lines blue in such a way that none of its finite regions has a completely blue boundary.
of the lines blue in such a way that none of its finite regions has a completely blue boundary.
Note: Results with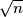 replaced by
replaced by 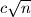 will be awarded points depending on the value of the constant
will be awarded points depending on the value of the constant  .
.
 , in any set of
, in any set of  lines in general position it is possible to colour at least
lines in general position it is possible to colour at least 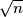 of the lines blue in such a way that none of its finite regions has a completely blue boundary.
of the lines blue in such a way that none of its finite regions has a completely blue boundary.Note: Results with
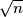 replaced by
replaced by 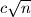 will be awarded points depending on the value of the constant
will be awarded points depending on the value of the constant  .
.  Školjka
Školjka  be an infinite sequence of positive integers. Prove that there exists a unique integer
be an infinite sequence of positive integers. Prove that there exists a unique integer  such that
such that 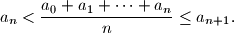
 has
has 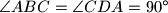 . Point
. Point  is the foot of the perpendicular from
is the foot of the perpendicular from  to
to  . Points
. Points  to
to  lie on sides
lie on sides  and
and  , respectively, such that
, respectively, such that  and
and 
 .
.  and
and  lie on side
lie on side  of acute-angled triangle
of acute-angled triangle  so that
so that 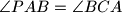 and
and 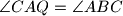 . Points
. Points  and
and  lie on lines
lie on lines  and
and 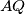 , respectively, such that
, respectively, such that  , and
, and 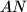 . Prove that lines
. Prove that lines  and
and 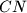 intersect on the circumcircle of triangle
intersect on the circumcircle of triangle