IMO Shortlist 1966 problem 13
Dodao/la:
arhiva2. travnja 2012. Let
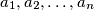
be positive real numbers. Prove the inequality
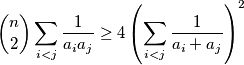
%V0
Let $a_1, a_2, \ldots, a_n$ be positive real numbers. Prove the inequality
$$\binom n2 \sum_{i<j} \frac{1}{a_ia_j} \geq 4 \left( \sum_{i<j} \frac{1}{a_i+a_j} \right)^2$$
Izvor: Međunarodna matematička olimpijada, shortlist 1966