IMO Shortlist 1966 problem 47
Dodao/la:
arhiva2. travnja 2012. Consider all segments dividing the area of a triangle

in two equal parts. Find the length of the shortest segment among them, if the side lengths
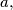
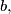

of triangle

are given. How many of these shortest segments exist ?
%V0
Consider all segments dividing the area of a triangle $ABC$ in two equal parts. Find the length of the shortest segment among them, if the side lengths $a,$ $b,$ $c$ of triangle $ABC$ are given. How many of these shortest segments exist ?
Izvor: Međunarodna matematička olimpijada, shortlist 1966