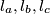
A line is drawn through the intersection point of altitudes of acute-angle triangles. Prove that symmetric images of with respect to the sides have one point in common, which lies on the circumcircle of
%V0
A line $l$ is drawn through the intersection point $H$ of altitudes of acute-angle triangles. Prove that symmetric images $l_a, l_b, l_c$ of $l$ with respect to the sides $BC,CA,AB$ have one point in common, which lies on the circumcircle of $ABC.$
Izvor: Međunarodna matematička olimpijada, shortlist 1967
Školjka 
is drawn through the intersection point 
of altitudes of acute-angle triangles. Prove that symmetric images 
of 
have one point in common, which lies on the circumcircle of 