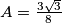
Prove that the product of the radii of three circles exscribed to a given triangle does not exceed times the product of the side lengths of the triangle. When does equality hold?
%V0
Prove that the product of the radii of three circles exscribed to a given triangle does not exceed $A=\frac{3\sqrt 3}{8}$ times the product of the side lengths of the triangle. When does equality hold?
Izvor: Međunarodna matematička olimpijada, shortlist 1968
Školjka 
times the product of the side lengths of the triangle. When does equality hold?