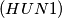
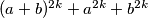
Let and be arbitrary integers. Prove that if is an integer not divisible by , then is divisible by
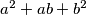
%V0
$(HUN 1)$ Let $a$ and $b$ be arbitrary integers. Prove that if $k$ is an integer not divisible by $3$, then $(a + b)^{2k}+ a^{2k} +b^{2k}$ is divisible by $a^2 +ab+ b^2$
Izvor: Međunarodna matematička olimpijada, shortlist 1969
Školjka 
Let 
and 
be arbitrary integers. Prove that if 
is an integer not divisible by 
, then 
is divisible by 