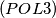
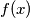
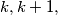
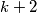
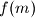
Given a polynomial with integer coefficients whose value is divisible by for three integers and . Prove that is divisible by for all integers
%V0
$(POL 3)$ Given a polynomial $f(x)$ with integer coefficients whose value is divisible by $3$ for three integers $k, k + 1,$ and $k + 2$. Prove that $f(m)$ is divisible by $3$ for all integers $m.$
Izvor: Međunarodna matematička olimpijada, shortlist 1969
Školjka 
Given a polynomial 
with integer coefficients whose value is divisible by 
for three integers 
and 
. Prove that 
is divisible by 