IMO Shortlist 1969 problem 63
Dodao/la:
arhiva2. travnja 2012. 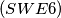
Prove that there are infinitely many positive integers that cannot be expressed as the sum of squares of three positive integers.
%V0
$(SWE 6)$ Prove that there are infinitely many positive integers that cannot be expressed as the sum of squares of three positive integers.
Izvor: Međunarodna matematička olimpijada, shortlist 1969