IMO Shortlist 1969 problem 65
Dodao/la:
arhiva2. travnja 2012. 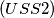
Prove that for
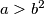
, the identity
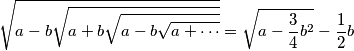
holds.
%V0
$(USS 2)$ Prove that for $a > b^2$, the identity $$\sqrt{a-b\sqrt{a+b\sqrt{a-b\sqrt{a+\cdots}}}}=\sqrt{a-\frac{3}{4}b^2}-\frac{1}{2}b$$ holds.
Izvor: Međunarodna matematička olimpijada, shortlist 1969