IMO Shortlist 1971 problem 17
Dodao/la:
arhiva2. travnja 2012. Prove the inequality
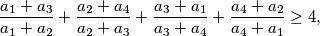
where

%V0
Prove the inequality
$$\frac{a_1+ a_3}{a_1 + a_2} + \frac{a_2 + a_4}{a_2 + a_3} + \frac{a_3 + a_1}{a_3 + a_4} + \frac{a_4 + a_2}{a_4 + a_1} \geq 4,$$
where $a_i > 0, i = 1, 2, 3, 4.$
Izvor: Međunarodna matematička olimpijada, shortlist 1971