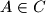
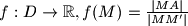
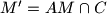
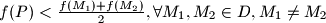
Let be the interior of the circle and let . Show that the function where , is strictly convex; i.e., where is the midpoint of the segment
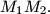
%V0
Let $D$ be the interior of the circle $C$ and let $A \in C$. Show that the function $f : D \to \mathbb R, f(M)=\frac{|MA|}{|MM'|}$ where $M' = AM \cap C$, is strictly convex; i.e., $f(P) <\frac{f(M_1)+f(M_2)}{2}, \forall M_1,M_2 \in D, M_1 \neq M_2$ where $P$ is the midpoint of the segment $M_1M_2.$
Izvor: Međunarodna matematička olimpijada, shortlist 1985
Školjka 
be the interior of the circle 
and let 
. Show that the function 
where 
, is strictly convex; i.e., 
where 
is the midpoint of the segment 