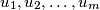
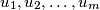
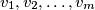
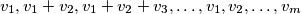
Let be vectors in the plane, each of length with zero sum. Show that one can arrange as a sequence such that each partial sum has length less than or equal to
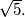
%V0
Let $u_1, u_2, \ldots, u_m$ be $m$ vectors in the plane, each of length $\leq 1,$ with zero sum. Show that one can arrange $u_1, u_2, \ldots, u_m$ as a sequence $v_1, v_2, \ldots, v_m$ such that each partial sum $v_1, v_1 + v_2, v_1 + v_2 + v_3, \ldots, v_1, v_2, \ldots, v_m$ has length less than or equal to $\sqrt {5}.$
Izvor: Međunarodna matematička olimpijada, shortlist 1988
Školjka 
be 
vectors in the plane, each of length 
with zero sum. Show that one can arrange 
such that each partial sum 
has length less than or equal to 