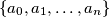
The set of real numbers satisfies the following conditions:
(i) (ii) for
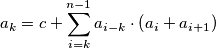
Prove that
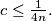
%V0
The set $\{a_0, a_1, \ldots, a_n\}$ of real numbers satisfies the following conditions:
(i) $a_0 = a_n = 0,$
(ii) for $1 \leq k \leq n - 1,$ $$a_k = c + \sum^{n-1}_{i=k} a_{i-k} \cdot \left(a_i + a_{i+1} \right)$$
Prove that $c \leq \frac{1}{4n}.$
Izvor: Međunarodna matematička olimpijada, shortlist 1989
Školjka 
of real numbers satisfies the following conditions: 
