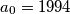
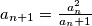
Let and for each nonnegative integer . Prove that is the greatest integer less than or equal to ,
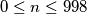
%V0
Let $a_{0} = 1994$ and $a_{n + 1} = \frac {a_{n}^{2}}{a_{n} + 1}$ for each nonnegative integer $n$. Prove that $1994 - n$ is the greatest integer less than or equal to $a_{n}$, $0 \leq n \leq 998$
Izvor: Međunarodna matematička olimpijada, shortlist 1994
Školjka 
and 
for each nonnegative integer 
. Prove that 
is the greatest integer less than or equal to 
, 