IMO Shortlist 1998 problem G7
Dodao/la:
arhiva2. travnja 2012. Let

be a triangle such that
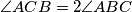
. Let

be the point on the side

such that
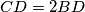
. The segment

is extended to

so that
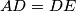
. Prove that
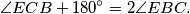
commentEdited by Orl.
%V0
Let $ABC$ be a triangle such that $\angle ACB=2\angle ABC$. Let $D$ be the point on the side $BC$ such that $CD=2BD$. The segment $AD$ is extended to $E$ so that $AD=DE$. Prove that
$$\angle ECB+180^{\circ }=2\angle EBC.$$
commentEdited by Orl.
Izvor: Međunarodna matematička olimpijada, shortlist 1998