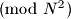
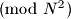
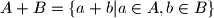
Let be a set of residues . Prove that there exists a set of residues such that contains at least half of all the residues .
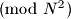
%V0
Let $A$ be a set of $N$ residues $\pmod{N^{2}}$. Prove that there exists a set $B$ of $N$ residues $\pmod{N^{2}}$ such that $A + B = \{a+b|a \in A, b \in B\}$ contains at least half of all the residues $\pmod{N^{2}}$.
Izvor: Međunarodna matematička olimpijada, shortlist 1999
Školjka 
be a set of 
residues 
. Prove that there exists a set 
of 
contains at least half of all the residues