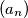
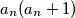
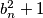
Prove that there exists two strictly increasing sequences and such that divides for every natural n.
%V0
Prove that there exists two strictly increasing sequences $(a_{n})$ and $(b_{n})$ such that $a_{n}(a_{n}+1)$ divides $b^{2}_{n}+1$ for every natural n.
Izvor: Međunarodna matematička olimpijada, shortlist 1999
Školjka 
and 
such that 
divides 
for every natural n.