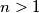
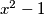
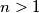
Given an integer , denote by the product of all positive integers less than and such that divides . For each , find the remainder of on division by .
%V0
Given an integer ${n>1}$, denote by $P_{n}$ the product of all positive integers $x$ less than $n$ and such that $n$ divides ${x^2-1}$. For each ${n>1}$, find the remainder of $P_{n}$ on division by $n$.
Izvor: Međunarodna matematička olimpijada, shortlist 2004
Školjka 
, denote by 
the product of all positive integers 
less than 
and such that 
. For each