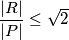
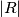
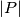
Let be a polygon that is convex and symmetric to some point . Prove that for some parallelogram satisfying we have where and denote the area of the sets and , respectively.
Proposed by Witold Szczechla, Poland
%V0
Let $P$ be a polygon that is convex and symmetric to some point $O$. Prove that for some parallelogram $R$ satisfying $P\subset R$ we have $$\frac{|R|}{|P|}\leq \sqrt 2$$ where $|R|$ and $|P|$ denote the area of the sets $R$ and $P$, respectively.
Proposed by Witold Szczechla, Poland
Izvor: Međunarodna matematička olimpijada, shortlist 2009
Školjka 
be a polygon that is convex and symmetric to some point 
. Prove that for some parallelogram 
satisfying 
we have 
where 
and 
denote the area of the sets