IMO Shortlist 2011 problem C7
Kvaliteta:
Avg: 0,0Težina:
Avg: 9,0 On a square table of 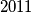 by
by 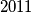 cells we place a finite number of napkins that each cover a square of
cells we place a finite number of napkins that each cover a square of  by
by  cells. In each cell we write the number of napkins covering it, and we record the maximal number
cells. In each cell we write the number of napkins covering it, and we record the maximal number  of cells that all contain the same nonzero number. Considering all possible napkin configurations, what is the largest value of
of cells that all contain the same nonzero number. Considering all possible napkin configurations, what is the largest value of  ?
?
Proposed by Ilya Bogdanov and Rustem Zhenodarov, Russia
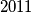 by
by 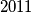 cells we place a finite number of napkins that each cover a square of
cells we place a finite number of napkins that each cover a square of  by
by  cells. In each cell we write the number of napkins covering it, and we record the maximal number
cells. In each cell we write the number of napkins covering it, and we record the maximal number  of cells that all contain the same nonzero number. Considering all possible napkin configurations, what is the largest value of
of cells that all contain the same nonzero number. Considering all possible napkin configurations, what is the largest value of  ?
?Proposed by Ilya Bogdanov and Rustem Zhenodarov, Russia
Izvor: Međunarodna matematička olimpijada, shortlist 2011
 Školjka
Školjka 
