IMO Shortlist 2011 problem G1
Let
be an acute triangle. Let
be a circle whose centre
lies on the side
. Suppose that
is tangent to
at
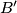
and
at
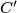
. Suppose also that the circumcentre
of triangle
lies on the shorter arc
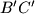
of
. Prove that the circumcircle of
and
meet at two points.
Proposed by Härmel Nestra, Estonia
%V0
Let $ABC$ be an acute triangle. Let $\omega$ be a circle whose centre $L$ lies on the side $BC$. Suppose that $\omega$ is tangent to $AB$ at $B'$ and $AC$ at $C'$. Suppose also that the circumcentre $O$ of triangle $ABC$ lies on the shorter arc $B'C'$ of $\omega$. Prove that the circumcircle of $ABC$ and $\omega$ meet at two points.
Proposed by Härmel Nestra, Estonia
Source: Međunarodna matematička olimpijada, shortlist 2011
Školjka 
