IMO Shortlist 2011 problem G7
Kvaliteta:
Avg: 0,0Težina:
Avg: 9,0 Let 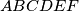 be a convex hexagon all of whose sides are tangent to a circle
be a convex hexagon all of whose sides are tangent to a circle  with centre
with centre  . Suppose that the circumcircle of triangle
. Suppose that the circumcircle of triangle 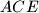 is concentric with
is concentric with  . Let
. Let  be the foot of the perpendicular from
be the foot of the perpendicular from  to
to  . Suppose that the perpendicular from
. Suppose that the perpendicular from  to
to  intersects the line
intersects the line 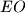 at a point
at a point  . Let
. Let  be the foot of the perpendicular from
be the foot of the perpendicular from  to
to  . Prove that
. Prove that 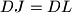 .
.
Proposed by Japan
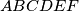 be a convex hexagon all of whose sides are tangent to a circle
be a convex hexagon all of whose sides are tangent to a circle  with centre
with centre  . Suppose that the circumcircle of triangle
. Suppose that the circumcircle of triangle 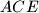 is concentric with
is concentric with  . Let
. Let  be the foot of the perpendicular from
be the foot of the perpendicular from  to
to  . Suppose that the perpendicular from
. Suppose that the perpendicular from  to
to  intersects the line
intersects the line 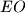 at a point
at a point  . Let
. Let  be the foot of the perpendicular from
be the foot of the perpendicular from  to
to  . Prove that
. Prove that 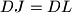 .
.Proposed by Japan
Izvor: Međunarodna matematička olimpijada, shortlist 2011
 Školjka
Školjka 
