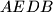
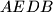
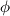
In a triangle , let and be the feet of the angle bisectors of angles and , respectively. A rhombus is inscribed into the quadrilateral (all vertices of the rhombus lie on different sides of ). Let be the non-obtuse angle of the rhombus. Prove that .
%V0
In a triangle $ABC$, let $D$ and $E$ be the feet of the angle bisectors of angles $A$ and $B$, respectively. A rhombus is inscribed into the quadrilateral $AEDB$ (all vertices of the rhombus lie on different sides of $AEDB$). Let $\phi$ be the non-obtuse angle of the rhombus. Prove that $\phi \leq \operatorname{max}\{ \angle BAC, \angle ABC \}$.
Školjka 
, let 
and 
be the feet of the angle bisectors of angles 
and 
, respectively. A rhombus is inscribed into the quadrilateral 
(all vertices of the rhombus lie on different sides of 
be the non-obtuse angle of the rhombus. Prove that 
.