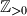
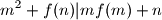
Let be the set of positive integers. Find all functions such that for all positive integers and .
%V0
Let $\mathbb{Z}_{>0}$ be the set of positive integers. Find all functions $f : \mathbb{Z}_{>0} \to \mathbb{Z}_{>0}$ such that $$
m^2 + f(n) | m f(m) + n
$$ for all positive integers $m$ and $n$.
Školjka 
be the set of positive integers. Find all functions 
such that 
for all positive integers 
and 
.