Školjka

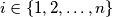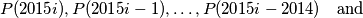
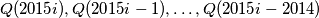
Let $n$ be a fixed integer with $n \ge 2$. We say that two polynomials $P$ and $Q$ with real coefficients are \emph{block-similar} if for each $i \in \{1, 2, \ldots, n\}$ the sequences \\$P(2015i), P(2015i - 1), \ldots, P(2015i - 2014) \quad \mbox{and}$\\ $Q(2015i), Q(2015i - 1), \ldots, Q(2015i - 2014)$\\\\ are permutations of each other. (a) Prove that there exist distinct block-similar polynomials of degree $n + 1$.\\ (b) Prove that there do not exist distinct block-similar polynomials of degree $n$. \begin{flushright}\emph{(Canada)}\end{flushright}