IMO Shortlist 2016 problem G6
Kvaliteta:
Avg: 0,0Težina:
Avg: 8,0Let  be a convex quadrilateral with
be a convex quadrilateral with 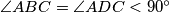 . The internal angle bisectors of
. The internal angle bisectors of 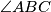 and
and  meet
meet  at
at  and
and  respectively, and meet each other at point
respectively, and meet each other at point  . Let
. Let  be the midpoint of
be the midpoint of  and let
and let  be the circumcircle of triangle
be the circumcircle of triangle 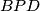 . Segments
. Segments  and
and 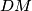 intersect
intersect  again at
again at  and
and  respectively. Denote by
respectively. Denote by  the intersection point of lines
the intersection point of lines 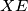 and
and 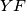 . Prove that
. Prove that 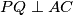 .
.
Izvor: https://www.imo-official.org/problems/IMO2016SL.pdf
 Školjka
Školjka 
