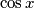
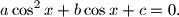
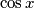
Let be real numbers. Consider the quadratic equation in Using the numbers form a quadratic equation in whose roots are the same as those of the original equation. Compare the equation in and for , , .
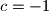
%V0
Let $a,b,c$ be real numbers. Consider the quadratic equation in $\cos{x}$ $$a \cos^2{x}+b \cos{x}+c=0.$$ Using the numbers $a,b,c$ form a quadratic equation in $\cos{2x}$ whose roots are the same as those of the original equation. Compare the equation in $\cos{x}$ and $\cos{2x}$ for $a=4$, $b=2$, $c=-1$.
Izvor: Međunarodna matematička olimpijada, shortlist 1959
Školjka 
be real numbers. Consider the quadratic equation in 
Using the numbers 
whose roots are the same as those of the original equation. Compare the equation in 
, 
, 
.