IMO Shortlist 1960 problem 5
Dodao/la:
arhiva2. travnja 2012. Consider the cube

(with face

directly above face
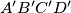
).
a) Find the locus of the midpoints of the segments

, where

is any point of

and

is any piont of
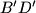
;
b) Find the locus of points

which lie on the segment

of part a) with

.
%V0
Consider the cube $ABCDA'B'C'D'$ (with face $ABCD$ directly above face $A'B'C'D'$).
a) Find the locus of the midpoints of the segments $XY$, where $X$ is any point of $AC$ and $Y$ is any piont of $B'D'$;
b) Find the locus of points $Z$ which lie on the segment $XY$ of part a) with $ZY=2XZ$.
Izvor: Međunarodna matematička olimpijada, shortlist 1960