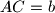
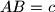
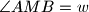
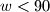
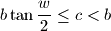
Construct a triangle if , and , where is the midpoint of the segment and . Prove that a solution exists if and only if In what case does the equality hold?
%V0
Construct a triangle $ABC$ if $AC=b$, $AB=c$ and $\angle AMB=w$, where $M$ is the midpoint of the segment $BC$ and $w<90$. Prove that a solution exists if and only if $$b \tan{\dfrac{w}{2}} \leq c <b$$ In what case does the equality hold?
Izvor: Međunarodna matematička olimpijada, shortlist 1961
Školjka 
if 
, 
and 
, where 
is the midpoint of the segment 
and 
. Prove that a solution exists if and only if 
In what case does the equality hold?