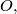
Prove that the sum of an odd number of vectors of length 1, of common origin and all situated in the same semi-plane determined by a straight line which goes through is at least 1.
%V0
Prove that the sum of an odd number of vectors of length 1, of common origin $O$ and all situated in the same semi-plane determined by a straight line which goes through $O,$ is at least 1.
Izvor: Međunarodna matematička olimpijada, shortlist 1973
Školjka 
and all situated in the same semi-plane determined by a straight line which goes through 
is at least 1.