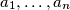
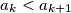
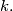
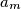
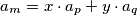
Let be an infinite sequence of strictly positive integers, so that for any Prove that there exists an infinity of terms , which can be written like with strictly positive integers and .
%V0
Let $a_{1}, \ldots, a_{n}$ be an infinite sequence of strictly positive integers, so that $a_{k} < a_{k+1}$ for any $k.$ Prove that there exists an infinity of terms $a_m$, which can be written like $a_m = x \cdot a_p + y \cdot a_q$ with $x,y$ strictly positive integers and $p \neq q$.
Izvor: Međunarodna matematička olimpijada, shortlist 1975
Školjka 
be an infinite sequence of strictly positive integers, so that 
for any 
Prove that there exists an infinity of terms 
, which can be written like 
with 
strictly positive integers and 
.